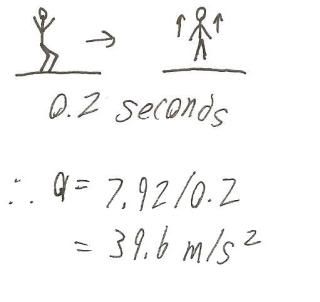Now, we all know that Physics in Anime is, for the most part, very unlike our own. However, often viewers will immediately dismiss their observations as impossible on our world, without any actual analysis. Now that’s not very scientific! So to save everyone else the time of doing this (and I am sure that everyone was, or was planning on doing this), I have done a small physical analysis of one such instance of Anime physics.
Now let us set the stage!
NOTE: I have several diagrams and equations in links. Please see them to better understand what I am talking about at that moment.
I believe that a very typical, and rather easy to analyze, situation is that of many characters’ extraordinary jumping ability. So let’s use Kenshin (of Rurouni Kenshin fame). Kenshin, off to save another would-be victim of Shishio, does not want Yahiko following him, afraid for his safety. As such, he decides to jump onto a nearby house, and continue onwards above the ground:

So, we have a house, let’s say 3 meters high, and Kenshin, who is, for argument’s sake, 1.6 meters tall (not that it actually matters for this problem), and weighs 50 kilograms. Now let’s draw a free body diagram of Kenshin at rest, and just to note, from now on we are considering Kenshin a point mass (as his mass is what matters, not his physical dimensions such as height).
If you don’t know what a point mass is, just imagine that Sanosuke, angry at Kenshin for running off without him, pounds Kenshin into a very small ball (don’t worry though, Kenshin is still alive. He IS Kenshin after all).
Just a small explanation of the force diagram: Normal Force is the force that is exactly equal and opposite the Gravitational Force (the force gravity is exerting on an object). It does not really matter that you understand what it is for this problem, so you can just ignore it if you do not understand.
Now, using Newton’s 2nd Law, let us calculate the current force on Kenshin (this is not necessary, but let’s do it anyway, just so that well all know what it is, as this equation will be needed later). Newton’s 2nd Law states that the Force (not from Star Wars, sorry fans) on an object is equal to the Mass of the object, times the Acceleration it is experiencing. Commonly it is written as F=ma. Just think of Fullmetal Alchemist (FMA), and you’ll remember the equation.
So let’s do the calculation of Kenshin at rest. Kenshin’s mass is 50 kg (kilograms). The current acceleration on Kenshin, though he is “at rest”, is the acceleration due to gravity, measured at 9.8 meters/(second*second), or 9.8 m/s^2 (9.8 meters per second squared). This is an acceleration that always exists on each one of us as we move around on the Earth. So even though you may be just sitting in your chair in front of your computer right now, you are in fact being “pushed down” by gravity, due to this acceleration.
So doing the calculation, we see that the Gravitational force on Kenshin is 490 Newtons down (Newtons are (kg*m)/s^2, kilogram meters per second squared). And since we know this, we also know that the Normal force on Kenshin is 490 Newtons up (equal and opposite force).
OK, so now we know what Kenshin is doing right now: he is getting ready to make the jump up to the house, but currently at rest. But how high is Kenshin getting ready to jump to? Sure the house is 3.0 m (meters) tall, but if Kenshin only jumped 3 m, that would be cutting it close, and may hit the house!

So let’s say that he will jump 3.2 m, just to be safe.
Another note: we will not worry about Kenshin’s horizontal distance that he needs to travel to make it onto the house, as it is not needed for what we are concentrating on. So when Kenshin jumps up, just imagine that someone pushes him in the air to make it to the house, or something to that effect.
Now let’s write down what we have, and what we need, so that we can figure out where to go from here.
So we know: that Kenshin’s mass is 50 kg, the distance he wishes to jump is 3.2 m, the time it will take him to make the jump is unknown (and not needed), his initial velocity (Vi) is unknown (this is what we want), his final velocity (Vf) is 0 m/s (as we are saying that his final velocity is the velocity when he reaches the top of his jump:

We are specifying that Kenshin’s final velocity (for now) is his movement at the point where he has stopped moving up, and is just about to come back down (If you do not understand this, don’t worry about it too much; it can be rather confusing at first). After that, Kenshin’s acceleration is –9.8 m/s^2 (gravity is negative as we have specified that “up” is the positive direction, so gravity is negative as it is pulling Kenshin “down”).
Phew!
We have the problem setup now! That is quite often the hardest part of a Physics problem. Once you have the problem setup, you can generally see what needs to be done, and simply plug things into equations.
Which is what we shall now do!
The first kinematics equation we shall use is this. Remember that Vf is final velocity, Vi is initial velocity, and that a is acceleration. “d”, is distance (sometimes “u” is also used), and the triangle thingy before it is “delta”, meaning the difference. Therefore “delta d” means the difference, or change in distance.
OK, so now let’s solve this. First let’s rearrange the equation, as we want to solve for Vi. And then we’ll plug in our numbers, and we get our answer! By our situation, Kenshin must be moving at approximately (that is what the = sign with a dot on top means, “approximately equal to”) 7.92 m/s the moment he leaves the ground if he wants to reach 3.2 m in height from his jump.
Another note: we are ignoring air resistance.
Wow! So 7.92 m/s! That is actually VERY fast. If you were running that fast, you’d finish a 100 meter sprint in just over 12 seconds!
But that is just the speed, what we REALLY want is the acceleration that Kenshin must go through to reach this speed. To REALLY understand what Kenshin must have done to move that fast, we need to find the force he must have used to jump. And we already know how to find that! That’s right, Fullmetal Alchemist to the rescue, F=ma!
So how do we find Kenshin’s acceleration? Well acceleration is the change in speed over an amount of time, hence the m/s^2 units: m/s is speed, so your change in speed over an amount of time would be velocity divided by the number of seconds that change took, therefore (m/s)/s = m/(s*s) = m/s^2.
So how do we find this? Well, we have to do a bit of assuming. We know that JUST before Kenshin left the ground, his vertical speed was 0 m/s, but JUST as he leaves the ground, his speed is 7.92 m/s. So when did his acceleration occur? Well, just as he began to push away from the ground. Which is a VERY small amount of time:
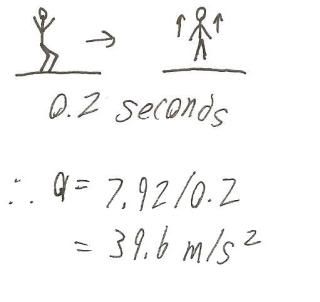
We are looking at the time of his legs extended from his crouching-ready-to-jump position to the moment his feet left the ground. So I’ll be generous here (over-estimate), and say that it took about 0.2 seconds. It probably took much less time, even though that is already a relatively small increment of time.
OK so, Kenshin accelerated 7.92 m/s in 0.2 seconds, therefore his acceleration is: 39.6 m/s^2.
Now THAT is VERY fast. If you accelerated at that speed, it would take you just over two seconds to do the 100 meter sprint! After 10 seconds of accelerating at this rate, you would be moving at a speed of 396 m/s! That’s 1425.6 km/h (or for those of you that use miles, 885.83 miles per hour)! This means that in ten seconds, you would go from not moving at all, to moving at a speed that would allow you to run across Canada in less than 4 hours!
I’m sure all of you can now appreciate the impossibilities that Kenshin must now be doing.
But let’s do one final calculation, this time using F=ma. By plugging in Kenshin’s mass (50 kg) and his acceleration (39.6 m/s^2), we find that the force he must have pushed off the ground with was 1980 N (Newtons).
Now this is harder to appreciate at face value, so let’s do a little calculation. Let’s say that you wanted to move the Kleenex box on your desk 10 centimeters in one second. That would mean that the acceleration would be 0.1 m/s^2. And let’s also say that you wanted to use the force that Kenshin used.
Therefore, we rearrange F=ma to m=F/a, and solve for the mass that the Kleenex box must be if you are going to move it with the same force, but at 0.1 m/s^2. This means that the box must weight a whopping 19 800 kg! That’s around the weight of four elephants (with an average elephant weighing at 5000 kg)!
Now this is harder to understand, but just think of it this way: the force at which Kenshin pushed off of the ground was also enough to move four elephants 10 centimeters in one second!
If it still isn’t getting through, don’t worry. Our other calculation is also just as valid, and unreal (doing the 100 meter sprint in just over two seconds).
Now I’m no biologist, but I’m PRETTY sure that these feats are impossible for humans. So therefore, BY SCIENCE (specifically Physics), we have PROVEN that some things that Anime does is impossible for a human on Earth. No “just saying”, no “it’s obvious”, we have the PROOF that it is.
So unless someone moves four elephants and gets it on video, I conclude that the jumping abilities of many anime characters are, by our current situation and standards on Earth, IMPOSSIBLE.
This has been Kastom for Anime Mythbuster.